Chapter 19: Selected Topics in Astrophysics

Equations Introduced or Used for this Topic:

 [latex]v_{esc} =\sqrt{2 G Mo / d_{o}}[/latex]                 [latex]Rs =\dfrac{2GM}{c^{2}}[/latex]

[latex]\Delta{E}_{p} = -\dfrac{Gm_{1}m_{2}}{d}[/latex]                   [latex]T = 2\pi\sqrt{r3 / G M}[/latex]    (Period of a Planet)

Where

M, Mo , m_1, m₂ is the Mass of the object, measured in Kilograms (kg)

v_esc   is the Speed that an object needs to permanently escape the gravitational field of a body, measured in Metres per Second (m/s)

r   is the Radius of the orbit, measured in Metres (m)

T  is the Period of Orbit, measured in Seconds (s)

G   is Newton’s Gravitational Constant, currently estimated to be 6.67408(31) x 10^-11 Nm²/kg²

∆V  is the Gravitational Potential of a mass measured in Joules per Kilogram (J/kg)

d or d_o   is the Distance away from the Mass Center of a Body (gravitational field) or the Distance between Mass Centers of Two Bodies (gravitational fields), measured in Metres (m)

v_esc  is the Escape Velocity needed to escape the body in metres per second (m/s)

r_o is the Distance away from the object’s mass center in Metres (m)

c is the speed of light in meters per second (2.9979 x 10⁸ m/s)

R_s   is the Schwarzschild radius or the event horizon of the black hole

T  is the period of orbit of the satellite measured in meters per second (m/s)

r  is the Orbital Distance between mass centers in Metres (m)

Nature Magazine Infographic of the week, March 8, 2019.

One of the challenges of placing satellites in orbit or in manned space missions is the problem of recent human ventures into space, from the planned and protested 2007 Chinese destruction of a satellite and of multiple accidental collisions of satellites and space debris.

As of July 2016, the United States Strategic Command tracked a total of 17, 852 artificial Earth orbit objects. As of January 2019 an estimated 129 million bits of debris were thought to be in orbit around the Earth.

As the numbers of these bits of debris increase, there exists the risk that the Earth’s orbit will become impassable due to potential collisions. Space debris is suspected or known to have damaged or destroyed over nine satellites to date.

The Shuttle Endeavour impact from debris is shown below.

INSERT SHUTTLE TRAFFIC JAM GRAPH

In the News – The European Space Agency is Sending a Robot to Hug Junk Out of Space: https://www.smithsonianmag.com/smart-news/european-space-agency-sending-robot-hug-junk-out-space-180973762/

19.1 Escape Velocity⁴

Escape velocity is the minimum speed needed for an object to permanently escape the influence of the gravitational force of a body, such as a planet, moon or star. The first such object launched from the Earth to achieve escape velocity did so on January 2nd, 1959, launched by the former USSR from the Baikonur Cosmodrome. It eventually reached an orbit around the Sun between the planets Earth and Mars.

INSERT IMAGE – ASK TERRY WHAT THE SILVER THING IS

The escape velocity equation can be algebraically derived from the conservation of mechanical energy combined with the equation for gravitational field strength. The use of the term “velocity” for this phenomenon is inaccurate, since only speed is required in any direction that does not intersect or return to the body it is escaping from. There are two conditions to look at when using the escape velocity equation: (i) the launch velocity is equal to the escape velocity, and (ii) the launch velocity is greater than the escape velocity.

If the launch velocity is equal to the escape velocity, then the object will continue to move away from the body it was launched from, continually slowing but never reaching 0 m/s as it moves to an infinite distance away. The equation defining this is:

[latex]v_{esc} =\sqrt{2 G Mo / r_{o}}[/latex]

Where:

v_esc =  is the Escape Velocity needed to escape the body in m/s

G  =  6.671 x 10^-11 Nm₂/kg₂

M_o  =  The Mass of the object in kg

r_o =  The Distance away from the object’s mass center in m

If the initial launch velocity is greater than the escape velocity, then the object’s final velocity as it escapes the body can be calculated using the following equation:

 E_kf = E_ki – E_kesc which simplifies to v_f²  =  v_i²  –  v_esc²    (all velocities are in m/s).

Using the conservation of mechanical energy, one is able to derive the escape velocity equation:

This looks like   E_ki  +  E_pi  =   E_pf   +  E_kf  where  (E_pf + E_kf) = 0 which means that

E_ki  +  E_pi  =  0

Further simplification is done by substitution, using the gravitational Potential Energy equation that is needed to escape a gravitational field for E_pi, specifically: E_pi = – Gm₁m₂/r

[latex]E_{ki} -\dfrac{Gm_{1}m_{2}}{r}= 0[/latex]

Since E_ki = 1/2 mv_i²

[latex]\dfrac{1}{2} mv_{i}^{2}  -\dfrac{Gm_{1}m_{2}}{r}= 0[/latex]

This changes to [latex]\dfrac{1}{2} mv_{i}^{2}  -\dfrac{Gm_{1}m_{2}}{r}= 0[/latex]

Cancelling the mass escaping the gravitational field and multiplying out the 1/2 fraction yields:

v_i²  =[latex]2\dfrac{Gm_{1}}{r}[/latex]

When removing the square and replacing the initial velocity (vi) with the escape velocity (v_esc), the equation in its final form is:

v_esc  =[latex]\sqrt{2 G M_{o} / r_{o}}[/latex]

This equation allows one to calculate the velocity of any object to escape the gravitational field of a body.⁵

Using the Conservation of Mechanical Energy:

E_kf = E_ki – ∆E_{k esc}

1/2 mv_f²   = 1/2 mv_i² – 1/2 mv_esc²   Cancel out the common mass and 1/2

We get v_f²   = v_i² – v_esc²

v_f² = (8400 m/s)² – (4900 m/s)²      v_f = 6820 m/s

19.2 The Size of Black Holes ^{6, 7, 8, 9, 10, 11}

The Schwarzschild radius (black hole event horizon) was calculated by Karl Schwarzschild in 1916 from his exact solution of Einstein’s General Relativity Equation.

This radius is given as                         R_s =[latex]\dfrac{2 G M}{c^{2}}[/latex]

The derivation of the black hole radius equation is found by using the escape velocity equation where v_esc  =  c.  In this case, the equation is solved for when light itself cannot escape the gravitational influence of the black hole.

The size of a black hole could be as small as an atom (primordial) and contain the mass of a large mountain¹². Other types of black holes are classified as stellar and could have a mass of twenty times the mass of our Sun [solar mass, M_o = (1.988 55 ± 0.000 25) x 1030 kg] and it is expected that many of these black holes exist in our own galaxy. The largest of the black holes bears the name supermassive¹³ and is expected to contain the mass of one million or more solar masses. Evidence has been found that indicates the center of every large galaxy has its own supermassive black hole. Our galaxy’s supermassive black hole has been estimated to contain 4.3 million solar mass and has been given the name Sagittarius A.

Black holes cannot be seen since light cannot escape from them. However they can be detected by observing the gas and stars that orbit them. Black holes have also been detected by the light that bends when passing by them and also from x-rays that are emitted by objects falling into the black hole.

The simple derivation of the Schwarzschild radius is done using the escape velocity equation and substituting the speed of light c for the escape velocity v_esc and replacing r_o with the Schwarzschild radius R_s.

    v_esc  =[latex]\sqrt{2 G M_{o} / r_{o}}[/latex]    then becomes    c  =[latex]\sqrt{2 G M_{o} / Rs}[/latex]

Squaring both sides of this equation yields:

c²  =[latex]\dfrac{2 G M_{o}}{R_{s}}[/latex]

Cross multiplying c²  and R_s  yields:

R_s =[latex]\dfrac{2 G M_{o}}{R_{s}}[/latex]

On April 10, 2019 scientists for 40 different nations released the first actual photo of a black hole¹⁴ culminating an effort of over 13 years. This photo (shown below) is of the supermassive black hole at the center of the Messier 87 galaxy and was accomplished using the Event Horizon Telescope (EHT)¹⁵.  The known details of this black hole include that it is located approximately 53.5 million light years away and has a mass of around 6.5 billion suns. The size of this black hole and its accretion disk (orbiting ring of hot matter) have an estimated diameter of 100 million km.

While some folks describe the black hole image as a shiny, glazed donut, astrophysicists note that it is slightly asymmetrical, due to the tilt of the accretion disk. Doppler shifts are also present in this image, where the bright side represents matter moving towards us and the darker side represents the matter moving away. Another challenge is that this image is only visible in certain ranges.  If observed too low or too high, the black hole image is obscured by the plasma surrounding it. The best wavelength to use to see through everything obscuring the black hole from view is that of microwaves at 230 GHz or 1.3 mm. This requirement necessitates a global collaboration to use telescopes in multiple settings on Earth to make the largest telescope possible. This allows for astrophysicists to get the resolution needed to see an object that has an angular dimension of 40 microarcseconds.^16, 17, 18

The image currently being shared results from the conversion of microwaves into visible wavelengths. If one were to attempt to see this using a visible light telescope, they would not succeed. The image below shows the location of this black hole at the center of M87.

19.3 Orbital Mechanics ^{19, 20, 21, 22}

Studies the motion of spacecraft orbiting objects in space, and the motion of any space-based object in an orbit, trapped in a gravitational field. For simplicity’s sake, we shall only consider circular orbits.

The physics used in working with circular orbits in space comes from gravitational force F_g and centripetal forceF_c. For this derivation, one must equate these two forces, specifically:

           F_g  =  F_c

[latex]\dfrac{Gm_{1}m_{2}}{d^{2}} =\dfrac{mv^{2}}{r}[/latex]

Since d and r are equivalent and m² and m are also equivalent, they can be cancelled out as common, which leaves us with:

[latex]\dfrac{Gm_{1}}{d} = v^{2}[/latex]

Since we will be solving for orbits, we will use the more common r for the radius of the orbit rather than d.

Calculating the speed of the satellite in orbit involves using:

[latex]v =\dfrac{2 π r}{T}[/latex] (distance) (time for one complete orbit)

Which for v² yields:[latex]v^{2} =\dfrac{4 π^{2}r^{2}}{T^{2}}[/latex]

Replacing v² in the equation:[latex]\dfrac{Gm_{1}}{d}= v^{2}[/latex]

Yields [latex]\dfrac{Gm_{1}}{d}\dfrac{4 π^{2}r^{2}}{T^{2}}[/latex]

Isolating T² yields:

[latex]T^{2} =\dfrac{4 π^{2}r^{3}}{Gm_{1}}[/latex]

Taking the square root of both sides leaves us with

[latex]T = 2π\sqrt{\dfrac{r^{3}}{Gm}}[/latex]

As you can see from the structure of this equation, it belongs to the family of simple harmonic equations used in Labs 4 and 5.

[latex]T =\sqrt{\dfrac{2πl}{g}} T =\sqrt{\dfrac{2 π m}{k}}[/latex]

19.4.1 Geosynchronous Satellites ^23, 24

Are satellites in geosynchronous orbit with an orbital period the same as the Earth’s rotation period.  Such a satellite returns to the same position in the sky after each sidereal day.  A special case of geosynchronous satellite is the geostationary satellite, which has a geostationary orbit – a circular geosynchronous orbit directly above the Earth’s equator.

Geostationary satellites appear to be fixed over one spot above the equator.  Receiving and transmitting antennas on the Earth do not need to track such a satellite.  These antennas can be fixed in place and are much less expensive than tracking antennas.  These satellites have revolutionized global communications, television broadcasting and weather forecasting, and have a number of important defense and intelligence applications.

One disadvantage of geostationary satellites is  the result of their high altitude: radio signals take approximately 0.25 of a second to reach and return from the satellite resulting in a small but significant signal delay.  This delay increases the difficulty of telephone conversation and reduces the performance of common network protocols such as TCP/IP but does not present a problem with non-interactive systems such as television broadcasts.  Another unusual feature is that time is distorted by the difference in the gravitational field strength on the Earth’s surface and at the geosynchronous orbit. This time difference amounts to 45 µs each day which means that data such as that used for GPS navigation must be updated on a regular basis to keep the information coming from these satellites accurate.

Another disadvantage of geostationary satellites is the incomplete geographical coverage, since ground stations at higher than roughly 60 degrees latitude have difficulty reliably receiving signals at low elevations.  Satellite dishes at such high latitudes would need to be pointed almost directly towards the horizon.  The signals would have to pass through the largest amount of atmosphere and could even be blocked by land topography, vegetation or buildings.

One of the greatest future prospects in using geosynchronous orbiting stations is that of placing the hub of a space elevator in that position.  China could build this by 2045, to enormous commercial benefit. This could see the cost of placing objects into geosynchronous orbit drop from around $22 000 per kilogram to $1.50 per kilogram.^25,26

The idea of a space elevator was first proposed in 1895 by a Russian scientist named Konstantin Tsiolkovsky, who, inspired by the Eiffel tower, speculated on constructing a similar tower to use as a base on Earth for a space elevator. The limitations of such an idea, which now appear to have been resolved by the development of carbon nanotube technology (CNT), have been in the design needs of the space cable itself.   Current production of carbon nanotubes is restricted to the lengths that can be produced in a laboratory, but as work in this area continues, CNT should produce a cable of the required length and diameter within a few decades. Space travel looks to be entering a new future with possibilities previously imagined in Science Fiction.

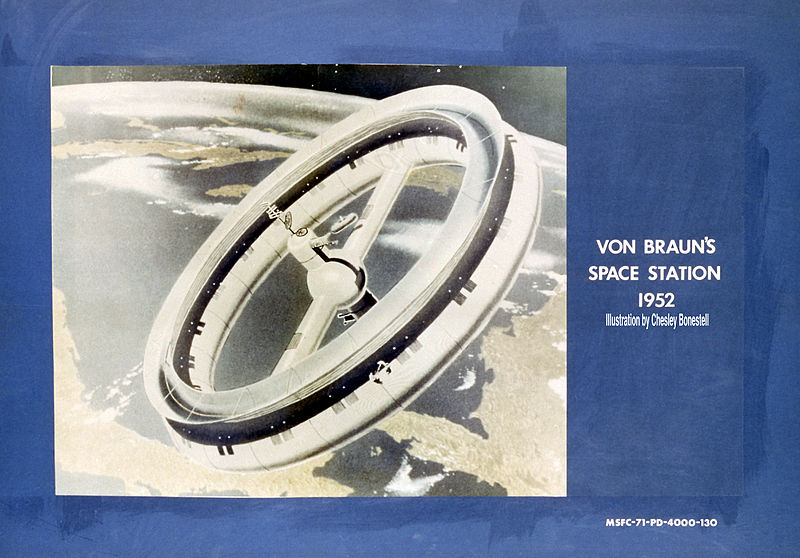
19.4.2 Artificial Gravity in Space  ²⁷

Weightlessness over extended periods of time has been found to have quite harmful effects on human physiology28, including loss of bone density (Spaceflight osteopenia), in which astronauts lose 1%+ of bone mass per month in space, risking irreversible skeletal damage and muscular atrophy.  Weightlessness also slows cardiovascular system functions, decreases the production of red blood cells, causes balance disorders, and weakens the immune system.  Lesser symptoms include fluid redistribution inside the body, disrupted vision, loss of taste, loss of body mass, nasal congestion, and sleep disturbance, among other problems.  Predicting problems in zero g environments, Wernher von Braun and Willy Ley in 1952 proposed a rotating space ship that could simulate gravity using centripetal force.  Their ship design included a 38 metre wheel that could rotate at 3 rpm, providing a 1/3 g gravity for a crew of 80.  One of the potential problems of rotating spaceships is that astronauts would experience less artificial gravity the closer they got to the center of the rotating ship.  This means that their head and feet would experience different gravities and would cause a Coriolis force for the human ear, which would cause dizziness, nausea and disorientation.

19.4.3 Measuring the Mass of the Sun²⁹

19.4.4 The Most Distant World Visited – Arrokoth ^{30, 31, 32}

On January 1st, 2019, NASA’s New Horizons spacecraft at a distance of 3500 km flew past 2014 MU₆₉ which accordingly is at a distance of 5.5 billion km (Wired Magazine) to 6.5 billion km (Nature Magazine) away from Earth, depending on the news source. Arrokoth (First Named as Ultima Thule) can be most accurately described as a rotating bowling pin 32 km long and 16 km wide. The complete download of data from the Horizon’s spacecraft is expected to take some 20 months, finishing by September 2020.

INSERT PICTURE – EARLY DATA RETURN ?jpeg

Question: Given consistent reports that Arrokoth takes a little over 298 years to orbit the Sun, what average orbital distance from the two different sources above is more accurate?

r = find T  =  2π[latex]\sqrt{r^{3} / G M}[/latex]  Isolate for r

T = 298 years or 9.4 x 10⁹ sr = ³[latex]\sqrt{T^{2}GM / 2π}[/latex]

G = 6.67 x 10^-11 Nm²/kg²

                                         r = ³[latex]\sqrt{(9.4\times10^{9}s)^{2} (6.67\times10^{-11} Nm^{2}/kg^{2})(1.9891\times10^{30}kg) / 2π}[/latex]

M = 1.9891 x 1030 kg

                                        [latex]r =_{3}\sqrt{2.97\times10^{30}  m^{3}}[/latex]

                                       r = 6.7 x 10¹² m or 6.7 billion km

Some known data about Arrokoth. Source. Nature 2019