Chapter 18: Centripetal Forces

Equations Introduced or Used for this Topic:
[latex]F_{c}=\dfrac{mv^{2}}{r}[/latex] [latex]a_{c}=\dfrac{v_{c}^{2}}{d}[/latex] [latex]v_{c}=\dfrac{d}{t}=\dfrac{2 πr}{T}[/latex]
[latex]F_{g}=\dfrac{Gm_{1}m_{2}}{d^{2}}[/latex] Ff = µN or “µmg” (simplest case)
Ff = µN where N = mg cosØ w = mg
Ffs = µsN Ffk = µkN Ffr = µrN
Where:
Fc is the Centripetal Force (Center Directed Force), measured in Newtons (N)
Ff is the Force of Friction measured in Newtons (N)
Ffs is the Force of Static Friction measured in Newtons (N)
Ffk is the Force of Kinetic Friction measured in Newtons (N)
FfR is the Force of Rolling Friction measured in Newtons (N)
Fg is the Gravitational Force of attraction measured in Newtons (N)
w is Weight (it is a Force) measured in Newtons (N)
N is the Normal Force measured in Newtons (N)
ac is the center directed acceleration, measured in Metres per Second squared (m/s2)
g or ag is the Acceleration due to Gravity and varies from 9.78 m/s2 (equator) to 9.83 m/s2 (North or South poles). The average value of gravity is taken to be either 9.80 m/s2 or 9.81 m/s
ag or g is the Gravitational Field Strength of a body measured in Metres/Second squared (m/s2) or Newtons per Kilogram (N/kg)
vc is the Speed of the object moving in a circular path, measured in Metres per Second (m/s)
m is the Mass of the object moving in a circular path, measured in Kilograms (kg)
m1 & m2 are the Masses of the two interacting bodies measured in Kilograms (kg)
r is the Radius of the circular path, measured in Metres (m)
d is the Distance away from the Mass Center of a Body (gravitational field) or the Distance between Mass Centers of Two Bodies (gravitational fields) measured in Metres (m)
µs µk & µr are Coefficients of Friction and are magnitudes without units
G is Newton’s Gravitational Constant currently estimated to be 6.67408(31) x 10-11 Nm2/kg2
T is the Period of the Simple Harmonic Oscillator, measuring the time it takes to make one complete motion measured in seconds (s)
18.1 Centripetal Force and Acceleration
[latex]F_{c}=\dfrac{mv^{2}}{r}[/latex] [latex]a_{c}=\dfrac{v_{c}^{2}}{r}[/latex] [latex]v_{c}=\dfrac{2 πr}{T}[/latex]
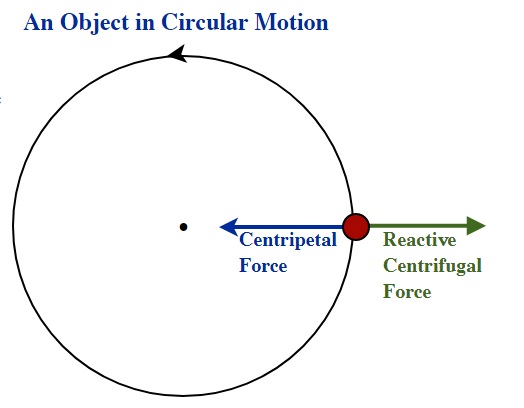
Centripetal Force is often confused with the term centrifugal force and it causes a number of problems for students learning the fundamental concepts of physics. To clarify this confusion, one needs to explore circular motion while applying Newton’s Laws of Motion.
Newton’s First Law states that every object will remain at rest or in uniform motion in a straight line unless compelled to change its state by the action of an external force. This means that if an object is moving in a circular path rather than in a straight line then there is some net force that is causing this to happen. For a circular path, this force will always be directed to the center of the circle. This feature gives rise to the name of this force, specifically a Center Directed Force or Centripetal Force (Fc).
Newton’s Second Law, when applied to motion in a circle, can be used to derive the centripetal force equation: Fnet = ma. This is done by replacing the linear acceleration with the value derived for centripetal acceleration4.
[latex]a_{c}=\dfrac{V^{2}}{r}[/latex] & Fc = mac combine to make [latex]F_{c}=\dfrac{mv^{2}}{r}[/latex]
Newton’s Third Law states that for every action (force) in nature there is an equal and opposite reaction. This can shed light on the idea of centrifugal force. To move an object in a circular path a center directed force is required. By Newton’s Third Law, there is a reactive force to the centripetal force which is called the reactive centrifugal force. One rarely sees reactive centrifugal force used in physics literature, however it is applied in some mechanical engineering settings where it has been used in laboratory devices such as the centrifuge.
EXAMPLE 18.1.1
How wide a radius of curvature will a 12 000 kg boat traveling at 36 km/h take on the water if it can only exert a centripetal force of 28000 N to make this turn?
[latex]F_{c}=\dfrac{Mv^{2}}{r}[/latex]
28000 N =[latex]\dfrac{(\text{12 000 kg})(\text{10 m/s})^{2}}{r}[/latex]
r = (1 200 000 kg m2/s2) ÷ 28000 N
r = 42.9 m or 43 m
EXAMPLE 18.1.2
What centripetal force must the tires of a 1600 kg truck exert on the road to turn a corner of radius 200 m at a speed of 60 km/h?
[latex]F_{c} =\dfrac{mv^{2}}{r}[/latex]
[latex]F_{c}=\dfrac{(\text{1600 kg})(\text{16.7 m/s})^{2}}{\text{200 m}}[/latex]
Fc = (444 400 m2/s2) ÷ 200 m
Fc = 2200 N
EXAMPLE 18.1.3
If you have a freeway that has a gentle curve of radius 600 m and the speed on this freeway is 120 km/h, how much time is required for a vehicle traveling at the speed limit to complete 1/4 of the turn?
First, the distance to travel 1/4 turn is 1/4 of a circular path of radius 600 m. Therefore:
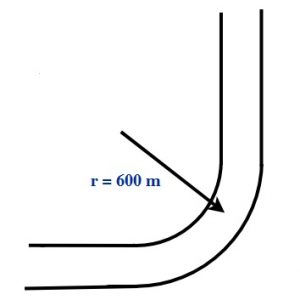
d = (1/4)(2 π r)
d = (1/4)(2 π 600 m) or 940 m
Now: how much time is needed to travel this distance traveling at 120 km/h?
[latex]v=\dfrac{d}{t}[/latex]
33.3 m/s =[latex]\dfrac{\text{940 m}}{t}[/latex]
t = 28 s
Centripetal Acceleration 5, 6, 7, 8, 9 is needed to move any object in a circular path. Similar to the unbalanced net force that is needed to cause an object to accelerate in a linear path, a centripetal net force will cause an object to accelerate to the center of the curved path that it moves in. This is different from linear acceleration that results in the magnitude of a change in speed or velocity. Centripetal acceleration can be as simple as the vector change in direction, such as the change occurring for the near circular orbit of Earth, always changing in direction but maintaining a near constant speed as it orbits the Sun. Other objects, such as comets having an elliptical orbit, have a combination of both linear and centripetal acceleration, and as such can be found to speed up and slow down while changing the direction of their motion. The physics in this textbook will only consider centripetal and linear acceleration and not analyze combinations of the two.
As explained previously, centripetal acceleration can be found by replacing both linear acceleration and force in Newton’s Second Law with centripetal acceleration (ac) and centripetal force (Fc). Doing this changes the equation of
F = ma to Fc = mac
Another variation of the equation for centripetal acceleration is one that comes from combining two centripetal force equations
Fc = [latex]\dfrac{mv^{2}}{r}[/latex]and Fc = mac
The result of doing this yields a centripetal acceleration equation of the form
[latex]ac =\dfrac{v^{2}}{r}[/latex]
EXAMPLE 18.1.4
Example 18.1.4 The NASA 20 g Centrifuge at the Ames Research Center10 can spin up to a 544 kg payload at 50 revolutions per minute (rpm). If the radius at which objects are spun is 8.84 m, how large a centripetal acceleration (measured in g’s) is reached when spinning at 50 rpm?

First: The distance traveled in a circle is
d = 2 π r
d = 2 π (8.84 m) or 55.5 m in one rotation.
Second: The time for one rotation
[latex]t =\dfrac{60 s}{\text{50 rotations}}[/latex] or 1.2 s to complete 1 rotation
Third: The rotational speed
[latex]v_{c}=\dfrac{d}{t}[/latex]
[latex]v_{c}=\dfrac{\text{55.5 m}}{1.2 s}[/latex] or 46.3 m/s
Fourth: The centripetal force compared to Newton’s second … (Fc = mac)
Fc = mac compared to Fc =[latex]\dfrac{mv^{2}}{r}[/latex]
[latex]ma_{c} =\dfrac{mv^{2}}{r}[/latex] cancelling the common mass yields [latex]a_{c} =\dfrac{v^{2}}{r}[/latex]
ac =[latex]\dfrac{(\text{46.3 m/s})^{s}}{\text{8.84 m}}[/latex]= 242 m/s2 which converts to 24.7 g’s
QUESTIONS 18.1 Centripetal Force
1. What centripetal force must the tires of a 1100 kg car exert on the road to turn a corner of radius 250 m at a speed of 90 km/h?
2. What centripetal force is needed to spin a 40 kg child at 1.4 m/s in a circle of radius 1.0 m?
3. A 120 kg skater using a frictional force of 1400 N makes a turn of radius 4.0 m. What was his speed?
4. What is the resulting radius of curvature if a 4000 kg boat traveling at 45 km/h exerts a centripetal force of 4200 N to make this turn?
5. A 1.2 kg object traveling with a speed of 5.6 m/s has a force of 7.4 N exerted on it perpendicular to the direction in which it is traveling. What is the radius of the circular path along which it moves?
6. What centripetal force does one need to exert on their 25 kg niece to spin her one complete spin in 3.0 s? (radius of curvature is 1.5 m. (This requires the calculation of rotational velocity.)
18.2 Centripetal Force & Roller Coasters
Equations Used:
[latex]F_{c}=\dfrac{mv^{2}}{r}[/latex] w = mg
The earliest looping roller coasters11 were given the name centrifugal railways, with the first of these built in 1843 in three cities in England. These rides were not actual roller coasters as we now think of the; rather they were a simple race down a slope into a loop using centripetal force to hold both the car and the passenger in the car with the remaining kinetic energy being enough to get the car back up to the other side. These rides did not gain much popularity and were only used for a short period. A sketch of the Manchester centrifugal railway is shown below.

The first vertical loop roller coaster resurfaced a little over 50 years later in 1895 at Coney Island, NY and was given the name the “Flip Flap Railway”. This ride survived for less than 7 years before being shut down due to the extreme g-forces averaging 12 gs on the riders. This caused riders significant discomfort and in a number of cases neck injuries from whiplash. The solution to salvaging this vertical loop ride was to design a tear drop shaped loop to reduce the g-forces in entering and leaving the loop.
The physics used to analyze these rides is to have the centripetal force remain greater than the force of gravity acting to pull the passengers and cars off the rails at the top of the loop.
EXAMPLE 18.2.1
What is the minimum speed of an amusement park loop-the-loop roller coaster that barely keeps you in your seat at the top of this loop? Assume a radius of rotation of 9.0 m.
We need to find the speed where Fc > w
[latex]\dfrac{mv^{2}}{r}[/latex] > mg … Cancelling the common mass leaves
[latex]\dfrac{v^{2}}{r}[/latex] > g or v2 > g r
Now … v2 > (9.80 m/s2)(9.0 m)
Which gives us v > 9.4 m/s
EXAMPLE 18.2.2
Will the passengers in a roller coaster be able to stay in their seats if the coaster slows to 45 km/h while at the top of a 15 m (radius) loop?
Check the speed to see if Fc > w
[latex]\dfrac{mv^{2}}{r}[/latex] > mg Cancelling the common mass leaves
[latex]\dfrac{v^{2}}{r}[/latex] > g or v2 > g r
(12.5 m/s)2 > (9.80 m/s2)(15 m)
156 m2/s2 > 147 m2/s2 Yes it is fast enough
EXAMPLE 18.2.3
What is the minimum speed of an amusement park pirate ship that just barely keeps you in your seat at the very top of the ride as they do a 360° loop? Assume a rotational radius of 12 m.
We need to find the speed where Fc ≈ w
[latex]\dfrac{mv^{2}}{r}[/latex] ≈ mg Cancelling the common mass leaves
[latex]\dfrac{v^{2}}{r}[/latex] ≈ g or v2 ≈ g r
Now v2 ≈ (9.80 m/s2)(12.0 m)
Which gives us v > 10.8 m/s
QUESTIONS 18.2 Centripetal Force and Roller Coasters
1. What is the minimum speed of the amusement park loop the loop roller coaster that barely keeps you in your seat at the top of this loop? Assume a radius of rotation of 12 m.
2. Will the passengers in a roller coaster be able to stay in their seats if the coaster slows to 20 km/h while at the top of a 14 m (radius) loop?
3. What is the minimum speed of an amusement park pirate ship that just barely keeps you in your seat at the very top of the ride as they do a 360° loop? Assume a rotational radius of 15 m.
18.3 Centripetal Force & Friction
Equations Used:
[latex]F_{c}=\dfrac{Mv^{2}}{r}[/latex] Ff = µN or “µmg” (simplest case)
Ff = µN where N = mg cosØ
Ffs = µsN Ffk = µkN Ffr = µrN
When we consider vehicles, objects or persons moving in a circular path on a road or a surface, it is frictional forces that allow this curved motion to occur. In this section, only horizontal curves will be analyzed, leaving banked curves to be covered in more advanced physics courses.
On horizontal surfaced curves the centripetal force allowing for curved motion to occur is friction. If we are looking at a vehicle such as a bus that needs to take a curve while driving along a road, the road conditions must be suitable to allow the friction between the tires and the road to be large enough to take this curve. The best friction for this is the static friction where the vehicle does not skid. The relationship for this looks like
Fc = Ffs
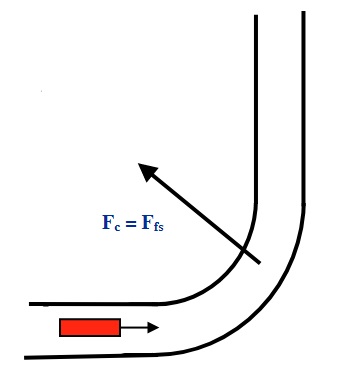
where the centripetal force allowing this bus to turn the corner is friction.
This equivalence equating the frictional force with the centripetal force works for all motion in a circular path where friction is the force that allows for these curved paths to be made.
The following examples all look at circular paths that are made using friction as the force to accomplish them.
EXAMPLE 18.3.1
What frictional force is needed by a snowboarder (mass = 55 kg) traveling at 42 km/h to take a turn of radius 10 m?
We need to find the friction where Ffk = Fc
[latex]Ff_{k}=\dfrac{mv^{2}}{r}[/latex]
[latex]Ff_{k}=\dfrac{(\text{55 kg})(\text{11.7 m/s})^{2}}{(\text{10 m})}[/latex]
Ffk = 750 N
EXAMPLE 18.3.2
What is the maximum speed that a 90 kg hockey player can take a 3.0 m radius curve where the frictional force is 4000 N?
We need to find the speed where Ffk = Fc
[latex]4000\text{ N}=\dfrac{(\text{90 kg})v_{2}}{\text{3.0 m}}[/latex]
[latex]v^{2}=\dfrac{(\text{4000 N})(\text{3.0 m})}{\text{90 kg}}[/latex]
v = 11.5 m/s
EXAMPLE 18.3.3
An 1800 kg truck travels around a level curve of radius 100 m. If the coefficient of friction between the truck tires and the road is µs = 0.65 what is the maximum speed this truck can take the curve without sliding?
We need to find the speed where Ffs = Fc
[latex]\text{μs mg cos Ø}\dfrac{mv^{2}}{r}[/latex] Cancel out the common mass, cos 0° = 1
[latex]μs g=\dfrac{v^{2}}{r}[/latex]
(0.65)(9.80 m/s2) =[latex]\dfrac{v^{2}}{\text{100 m}}[/latex]
v2 = (0.65)(9.80 m/s2)(100 m)
v = 25.2 m/s
QUESTIONS 18.3 Centripetal Force and Friction
1. What frictional force is needed by a downhill skier (mass = 65 kg) traveling at 80 km/h to take a turn of radius 12 m?
2. What frictional force is needed for a 1400 kg pickup to take a 200 m radius turn at 90 km/h?
3. What is the maximum speed at which a 1500 kg pickup can take a 50 m radius curve where the frictional force is 4200 N?
4. What frictional force is needed for a 1200 kg car to take a 150 m radius turn at 70 km/h?
5. A 1200 kg truck travels around a level curve of radius 50 m. If the coefficient of friction between the truck tires and the road is μ = 0.95 what is the maximum speed at which this truck can take the curve without sliding?
6. A downhill skier in a race enters a level curve traveling at 85 km/h. What coefficient of friction is acting if the skier manages to make a curve that has a radius of 11 m?
7. An 1100 kg convertible is traveling around a level curve of radius 60 m. If the coefficient of friction between the convertible tires and the road is μs = 0.75, will the convertible safely make this curve if traveling at 90 km/h? What should be the maximum posted speed for this curve, if ICBC expects an average coefficient of friction to be μs = 0.45?
18.4 Centripetal Force & Orbits 12, 13, 14, 15
Equations Used:
[latex]F_{c} =\dfrac{mv^{2}}{r}[/latex] [latex]F_{g}=\dfrac{Gm_{1}m{2}}{d^{2}}[/latex]
In 1609 and 1619 Johannes Kepler (1571-1630), building on the heliocentric concepts of Nicolas Copernicus (1473-1543) and the detailed telescopic data of Tycho Brahe (1546-1601), was able to arrive at three geometric equations that described the orbital motion of planets in a sun-centered solar system. His journey to arrive at these three laws is a complicated one that had him being expelled from one country due to his refusal to convert to Catholicism from his Calvinistic Christian upbringing, personal fights with Tycho Brahe in his desire to use Brahe’s data, all three children coming down with smallpox from his first wife (who died at an early age from Hungarian Spotted Fever), his first three children from his second marriage all dying in childhood, and his mother being imprisoned for fourteen months as a suspected witch. Making sense of Kepler’s writings requires one to know that in this time, there was no clear distinction between astronomy and astrology and that religion played a significant part in his writings.
Kepler himself believed that he had revealed God’s geometrical plan for the universe and they were written in such a manner that they fit the earlier Greek conceptions of the five platonic solids that were thought to be the building blocks of the universe. Kepler revealed the sketch of his model in an earlier book, “Mysterium Cosmographicum” (The Cosmographic Mystery, 1597). In this book Kepler defended Tycho Brahe against the then claims of plagiarism. Brahe allowed Kepler access to only his measurements of the planet Mars which was enough for Kepler to arrive at his three laws of planetary motion.
Kepler’s three laws of planetary motion16, 17 can be stated as follows:
• All planetary orbits are elliptical in shape and have the sun as one of the two foci. (For a circular orbit both foci are the same and at the center of the circle). (The Law of Orbits)
• If one were to draw a line from the center of the sun to the center of the orbiting planet, then the area swept out by the planet in orbit for any given amount of time will equal the area swept out by the same planet in any given equal amount of time, no matter where the planet is in in that orbit. (The Law of Areas)
• If one were to take the squares of the period of motion of any planet and divide it by the cube of the average distance of the planet to the sun, then one will arrive at the same constant of proportionality. (The Law of Periods)
While the paradigms grounding Kepler’s work are rooted in Greek philosophy and are only useful as historical background, his laws of motion still can be used to accurately describe the motion of any planet or satellite.
 One can see from the equations of the periods of simple harmonic oscillators shown to the right, that Kepler’s Third Law fits well with the other equations showing the simple harmonic motion of both the pendulum and Hooke’s Law for springs.
One can see from the equations of the periods of simple harmonic oscillators shown to the right, that Kepler’s Third Law fits well with the other equations showing the simple harmonic motion of both the pendulum and Hooke’s Law for springs.
Isaac Newton’s analysis of the acceleration of the Moon in its orbit around the Earth compared to the acceleration of objects on the Earth gave him the insight that the Moon orbited the Earth in a circular path due to the force of gravity that exists between them. The evidence for this came from combining Newton’s Universal Law of Gravitation with Centripetal Force to match the conclusion reached by Kepler in his Law of Periods for all planets. Newton was therefore able to arrive at a theoretical explanation for all known planetary data describing their orbits.
In this section, we equate Newton’s Universal Law of Gravitation with Centripetal Force to analyze the orbits of all satellites and planets by equating:
[latex]F_{c} =\dfrac{mv^{2}}{r}[/latex] [latex]F_{g}=\dfrac{Gm_{1}m{2}}{d^{2}}[/latex]
The following examples show how to equate these two:
EXAMPLE 18.4.1
Calculate the orbital speed of a satellite 280 km above the surface of the Earth.
Fc = Fg
[latex]\dfrac{mv^{2}}{r}=\dfrac{Gm_{1}m{2}}{d^{2}}[/latex] cancel out the common mass and distance
(radius of orbit = distance to Earth’s center)
[latex]v^{2} =\dfrac{Gm_{1}}{d}[/latex]
[latex]v^{2}=\dfrac{(6.67\times10^{-11}Nm^{2}/kg^{2})(5.974\times10^{24}kg)}{280 km + 6.371\times10^{6}m}[/latex]
[latex]v^{2}=\dfrac{3.98\times10^{14}Nm^{2}/kg}{6.651\times10^{6} m}[/latex]
v = 7740 m/s
Note: For these problems, the orbiting mass in the centripetal force equation is generally taken to be the secondary mass in Newton’s Universal Gravitation equation and the radius of orbit (r) is also the distance between the center of the mass and the planet.
EXAMPLE 18.4.2
What is the centripetal force needed to move the planet Venus in its current orbit around the Sun? Compare this with the gravitational force that exists between Venus and the Sun. (Venus takes 224.7 Earth days to orbit the Sun)
First: The distance travelled for one orbit is
d = 2 π r
d = 2 π (1.082 x 1011 m)
d = 6.80 x 1011 m in one orbit
Second: The time for one orbit
t = (224.7 days)(24 h/day)(3600 s/h)
t = 1.94 x 107 s
Third: The orbital speed
[latex]v_{c}=\dfrac{d}{t}[/latex]
[latex]v_{c}=\dfrac{6.80\times10^{11}m}{1.94\times10^{7}s}[/latex]
[latex]v_{c} = 3.50\times10^{4}\text{ m/s}[/latex]
Fourth: The centripetal force
[latex]F_{c}=\dfrac{mv^{2}}{r}[/latex]
[latex]F_{c}\dfrac{(4.869\times10^{24}kg)(3.50\times10^{4}m/s)^{2}}{1.082\times10^{11}}[/latex]
[latex]F_{c}=\dfrac{(4.869\times10^{24}kg)(3.50\times10^{4} m/s)^{2}}{1.082\times10^{11}m}[/latex]
[latex]F_{c} = 5.52\times10^{22}\text{ N}[/latex]
Comparing this to the gravitational force of attraction between the Sun and Venus
[latex]F_{g}=\dfrac{Gm^{1}m^{2}}{d^{2}}[/latex]
[latex]F_{g}\dfrac{(6.67\times10^{-11}Nm^{2}kg^{2})(1.9891\times10^{30}kg)(4.869\times10}{1.082 x 10^{11}}[/latex]
[latex]F_{g} =5.52\times10^{22}\text{ N}[/latex]
QUESTIONS 18.4 Centripetal Force and Orbits
1. Calculate the orbital speed of the moon as it orbits the earth.
2. What should be the orbital speed of a satellite 300 km above the surface of the Earth?
3. What is the centripetal force needed to move the Earth in its current orbit around the sun? Compare this with the gravitational force that exists between the Earth and the sun?
4. What is the centripetal force needed to move the planet Mercury in its current orbit around the Sun? Compare this with the gravitational force that exists between Mercury and the Sun. (Mercury takes 87.97 Earth days to orbit the Sun.)
5. What is the centripetal acceleration of the Moon directed towards Earth as it orbits the Earth? How does this compare to the average strength of the Sun’s gravity at the Moon’s orbit?
6. What is the centripetal acceleration of the Earth towards the Sun as it orbits the Sun? How does this compare to the strength of the Sun’s gravity at the Earth’s orbit?
QUESTIONS 18.5.1 The Mass of the Galaxy
Most of the stars in our galaxy, of which our sun is a member (the “Milky Way”) are concentrated in an assembly about 100 000 light years across, having a shape much like that of a fried egg. Our Sun is about 30 000 light years from the center of the galaxy and revolves around it in a period of around 225 million years.

Question: What is the approximate mass of our galaxy and how many stars of roughly the mass of our sun would this be equivalent to? (This data is continually being updated.)
QUESTIONS 18.5.2 The Rotor
The Rotor is an amusement park ride designed by Ernst Hoffmeister in the late 1940s and first operated at the 1949 Octoberfest. The Rotor is a large upright barrel rotated at speeds that create a Normal Force equivalent to almost 4 gs, that pins riders to the interior wall of the ride. Once the ride has attained full rotational speed, the floor is dropped down and at the end of the ride cycle the drum slows down allowing the riders to slowly slide down the wall to the dropped floor.
The Physics used to analyze this ride is Ff = Fg or the friction which equals the weight of the rider or
μN = mg.
The Normal Force can be found by the centripetal force that acts on the rider
[latex]F_{c}=\dfrac{mv^{2}}{r}[/latex] = N
Combining all the parts of the needed equation yields: μ [latex]\dfrac{mv^{2}}{r}[/latex]

Question: Given the rotor has a radius of about 2.4 m, calculate the rotational speed of the rider in the rotor for a projected coefficient of friction μs = 0.30 needed to prevent riders sliding down the wall during the ride.
REFERENCES
1. Mechanical Universe – Episode 9 – Moving in Circles: https://www.youtube.com/watch?v=MSUkQXA5_bU
2. A-Level Physics Tutor: http://www.a-levelphysicstutor.com/index-mech.php
3. Article to Read – ‘True polar wander’ may have caused ice age: https://phys.org/news/2018-11-true-polar-ice-age.html
4. Extra Help – Visual understanding of centripetal acceleration formula: https://www.khanacademy.org/science/ap-physics-1/ap-centripetal-force-and-gravitation/centripetal-acceleration-ap/v/visual-understanding-of-centripetal-acceleration-formula

