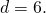Midterm 1 Preparation
This chapter contains several sections that will help you prepare for the midterm exam:
| Midterm 1 Composition Midterm 1 Review Midterm Sample A Midterm Sample B Midterm Sample C Midterm Sample D Midterm Sample E |
Midterm One: Composition
The first midterm will be composed of fifteen questions covering chapters 1 to 4.
Twelve questions will be algebra questions, and three questions will be word problems.
- Questions 1–4 will be drawn from Chapter 1: Algebra Review and Chapter 2: Linear Equations
- 1.1 Integers
- 1.2 Fractions
- 1.3 Order of Operations
- 1.4 Properties of Algebra
- 1.5 Terms and Definitions
- 2.1 Elementary Linear Equations
- 2.2 Solving Linear Equations
- 2.3 Intermediate Linear Equations
- 2.4 Fractional Linear Equations
- 2.5 Absolute Value Equations
- 2.6 Working with Formulas
- Questions 5–8 will be drawn from Chapter 3: Graphing
- 3.1 Points and Coordinates
- 3.2 Midpoint and Distance Between Points
- 3.3 Slopes and Their Graphs
- 3.4 Graphing Linear Equations
- 3.5 Constructing Linear Equations
- 3.6 Perpendicular and Parallel Lines
- Questions 9–12 will be drawn from Chapter 4: Inequalities
- 4.1 Solve and Graph Linear Inequalities
- 4.2 Compound Inequalities
- 4.3 Linear Absolute Value Inequalities
- 4.4 2D Inequality and Absolute Value Graphs
-
Questions 13–15 will be drawn from:
- 1.6 Unit Conversion Word Problems
- 2.7 Variation Word Problems
- 3.7 Numeric Word Problems
- 4.5 Geometric Word Problems
Students will be allowed to use MATQ 1099 Data Booklets for both Midterms and Final Exam.
Midterm One Review Questions
Questions 1–12 are for review only and will not show up on exams.
 (true or false)
(true or false)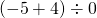
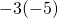
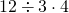
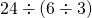
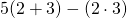
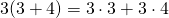 (true or false)
(true or false)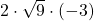
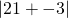
-
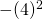
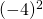
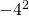
Questions similar to 13–20 will show up on exams.
- Evaluate:
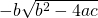 if
if 
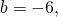 and
and  .
.
Chapter 2: Linear Equations (Exam Type Questions)
- Solve for
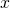 in the equation
in the equation 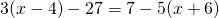 .
. - Isolate the variable
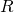 in the following equation:
in the following equation: 
- Solve for
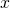 in the equation
in the equation 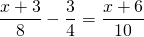
Chapter 3: Graphing (Exam Type Questions)
- Find the equation of the horizontal line that passes through the point

- Find the equation that has a slope of
 and passes through the point
and passes through the point 
- Find the equation of the line passing through the points
 and
and 
- Graph the relation
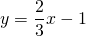 .
. - Find the distance between the data points
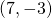 and
and 
- Find the midpoint between the data points
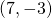 and
and 
Chapter 4: Inequalities (Exam Type Questions)
Questions 23–26 are for review only and will not show up on exams.
- 0 is a whole number (true or false)
- The elements of the set of integers are elements of the set of rational numbers (true or false)
- If
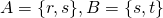 and
and 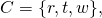 then
then 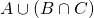 equals what?
equals what? 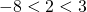 (true or false)
(true or false)
Questions similar to 27–37 will show up on exams.
For questions 27 to 30, find each solution set and graph it.
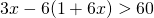
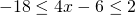
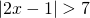

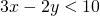
- Graph
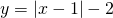 .
.
Chapter 1–3 Word Problems (Exam Type Questions)
- Find two numbers such that 6 times the larger number plus 2 times the smaller is 38, and 4 times the larger minus twice the smaller is 12.
- Karl is going to cut a 36 cm cable into 2 pieces. If the first piece is to be 5 times as long as the second piece, find the length of each piece.
- Kyra gave her brother Mark a logic question to solve: if she has 14 coins in her pocket worth
 and if the coins are only dimes and quarters, how many of each kind of coin does she have?
and if the coins are only dimes and quarters, how many of each kind of coin does she have? - Find two consecutive even integers such that their sum is 10 less than the first integer.
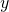 varies jointly with
varies jointly with 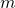 and the square of
and the square of 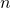 and inversely with
and inversely with 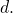 If
If 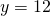 when
when 
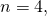 and
and 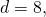 find the constant
find the constant 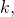 then use
then use 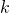 to find
to find 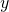 when
when 
 and
and