Even though the benzene ring was represented as a hexagon ring with alternating doubles and single bonds, the property and reactivity of benzene have nothing to do with usual alkenes (double bonds). Instead, the benzene ring shows special reactivity and stability.

First, the most common reaction of benzene is substitution, instead of the addition reaction for alkenes.

Secondly, the heat of hydrogenation data indicates the unique thermostability of the benzene ring. The heat of hydrogenation for cyclohexene with 1mol of hydrogen to cyclohexane is -120 kJ/mol, and that of 1,3-cyclohexadiene with two equivalents of hydrogens is -232 kJ/mol. The ΔH of the cyclohexadiene is roughly twice that of cyclohexene, with 8 kJ/mol less. The difference can be explained due to the stability of conjugated diene, as we learned in section 3.3.

According to the above trend, the calculated ΔH of benzene (if benzene does behave as “cyclohexatriene”) should be about -360kJ/mol. However the actual amount is -208 kJ/mol, way less than the calculated value, and it indicates that benzene is much more stable than cyclohexatriene. Such an energy difference of 152 kJ/mol indicates that the benzene ring is much more stable than a “regular” conjugated system. The special stability of benzene can be explained in two ways: resonance theory and molecular orbital theory.

Resonance Structures of Benzene

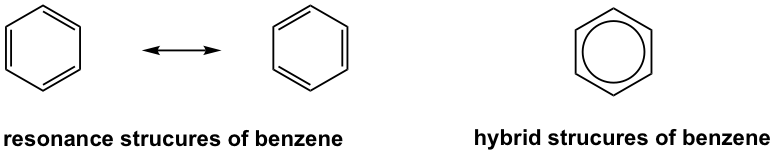
According to resonance theory, there are two equivalent resonance contributors for the structure of benzene. A hybrid structure (represented by a circle inside the hexagon) that has neither pure sing bonds nor pure double bonds represents the actual structure of benzene in a better way. Further experiment evidence shows that all of the carbon-carbon bonds in benzene are of the same length, so they are all the same type of bond with the same bond order, and they are neither pure single bonds nor pure double bonds. All the bond angles in the benzene ring are 120°, therefore all the carbons are in sp² hybridization with an unhybridized 2p orbital for each. The six carbon atoms construct a planar ring, and all the 2p orbitals are parallel to each other. Rather than form three localized π bonds, the six 2p orbitals overlap all together and form a big delocalized π bond, which explains the unique stability of the benzene ring. The energy difference of 152 kJ/mol between benzene and hypothetical 1,3,5-cyclohexatriene therefore can be called the resonance stabilization energy.

The molecular orbital theory then applies to understanding more about the delocalized π bond.

Molecular Orbital Theory

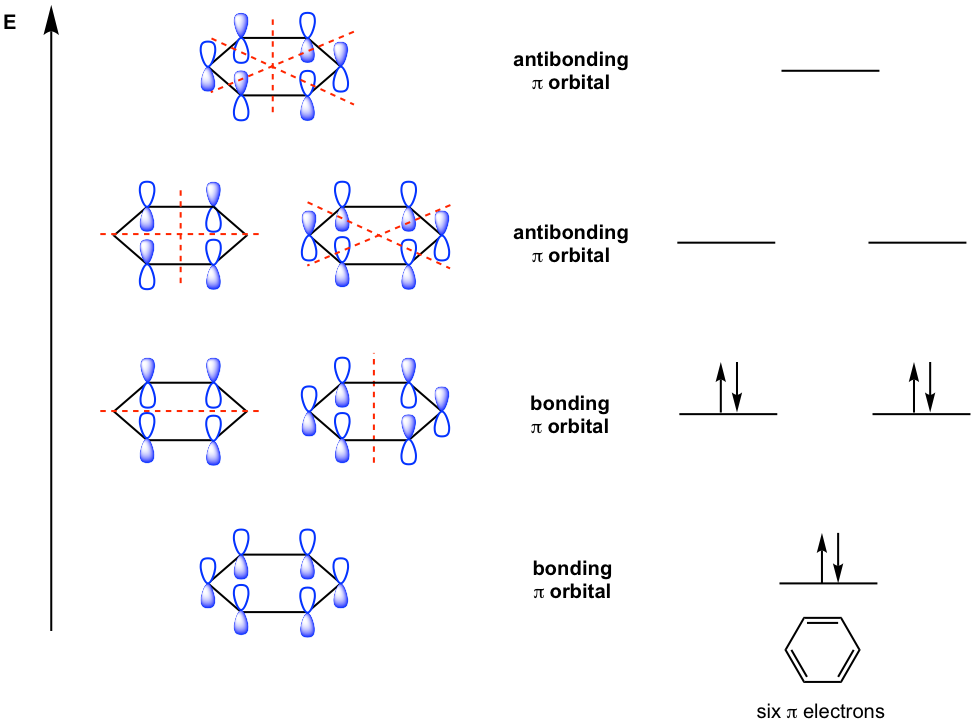
With six 2p orbitals combined, there are six π molecular orbitals, as shown in Fig. 4.2d. Three of them are bonding π MOs, and the other three are antibonding π MOs. Please note that one bonding π MO is in the lowest energy level, and the other two degenerate bonding π MOs are at the 2^nd high energy level and both have one nodal plane. Similarly, there are two antibonding π MOs at the next energy level with two nodal planes for each one. The antibonding π MO at the highest energy level has three nodal planes. With a total of six π electrons for benzene, all the electrons occupy the bonding MOs, which accounts for the stability of benzene.