Midterm 1: Version C Answer Key
- [latex]\begin{array}{l} \\ \\ \\ \\ -(4)-\sqrt{4^2-4(4)1} \\ \\ -4-\sqrt{16-16} \\ \\ -4 \end{array}[/latex]
- [latex]\begin{array}{rrrrrrrcrrrr} \\ \\ \\ \\ \\ &2x&-&8&+&8&=&3&-&7x&-&21 \\ +&7x&&&&&&&+&7x&& \\ \hline &&&&&\dfrac{9x}{9}&=&\dfrac{-18}{9}&&&& \\ \\ &&&&&x&=&-2&&&& \end{array}[/latex]
- [latex]\phantom{1}[/latex]
[latex]\left(A=\dfrac{h}{B+b}\right)(B+b) \\[/latex]
[latex]\begin{array}{rrrrrrr} \\ \\ \\ \\ \\ \dfrac{A}{A}(B&+&b)&=&\dfrac{h}{A}&& \\ \\ B&+&b&=&\dfrac{h}{A}&& \\ &-&b&&&-&b \\ \hline &&B&=&\dfrac{h}{A}&-&b \end{array}[/latex] - [latex]\phantom{1}[/latex]
[latex]\left(\dfrac{x}{15}-\dfrac{x-3}{3}=\dfrac{1}{5}\right)(15) \\[/latex]
[latex]\begin{array}{rrcrrrl} x&-&5(x&-&3)&=&3(1) \\ x&-&5x&+&15&=&\phantom{-1}3 \\ &&&-&15&&-15 \\ \hline &&&&\dfrac{-4x}{-4}&=&\dfrac{-12}{-4} \\ \\ &&&&x&=&3 \end{array}[/latex] - [latex]x=-2[/latex]
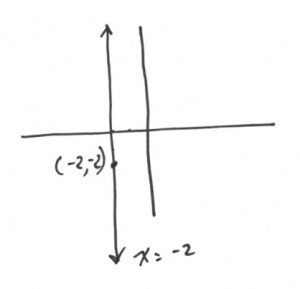
- [latex]\begin{array}{rrl} \\ \\ \\ \\ y&=&mx+6 \\ \therefore y&=&\dfrac{2}{3}x-3 \\ \\ \text{or } 3y&=&2x-9 \\ 0&=&2x-3y-9 \\ \end{array}[/latex]
- [latex]\begin{array}{ll} \begin{array}{rrl} \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ &&\textbf{1st slope} \\ m&=&\dfrac{\Delta y}{\Delta x} \\ \\ m&=&\dfrac{4--6}{-1-14} \\ \\ m&=&\dfrac{10}{-15} \\ \\ m&=&-\dfrac{2}{3} \\ \\ &&\textbf{Now:} \\ m&=&\dfrac{\Delta y}{\Delta x} \\ \\ -\dfrac{2}{3}&=&\dfrac{y-4}{x--1} \end{array} & \hspace{0.25in} \begin{array}{rrrrrrrlrr} \\ \\ \\ \\ \\ &&&-2(x&+&1)&=&\phantom{-}3(y&-&4) \\ &&&-2x&-&2&=&\phantom{-}3y&-&12 \\ +&&&-3y&+&12&&-3y&+&12 \\ \hline &-2x&-&3y&+&10&=&0&& \\ \text{or}&2x&+&3y&-&10&=&0&& \\ \\ &&&&&y&=&-\dfrac{2}{3}x&+&\dfrac{10}{3} \end{array} \end{array}[/latex]
-
[latex]2x-y=-2[/latex] [latex]x[/latex] [latex]y[/latex] 0 2 −1 0 −2 −2 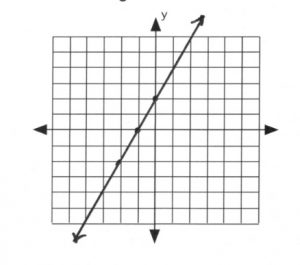
- [latex]\begin{array}{rrrrrrr} \\ \\ \\ \\ \\ 0&\le &2x&+&4&<&8 \\ -4&&&-4&&&-4 \\ \hline \dfrac{-4}{2}&\le &&\dfrac{2x}{2}&&<&\dfrac{4}{2} \\ \\ -2&\le &&x&&<&2 \end{array}[/latex]

- [latex]\begin{array}{rrrrrrrrrrr} \\ \\ y&-&1&>&3&\hspace{0.25in} \text{or}\hspace{0.25in}&y&-&1&<&-3 \\ &+&1&&+1&&&+&1&&+1 \\ \hline &&y&>&4&\hspace{0.25in} \text{or}\hspace{0.25in}&&&y&<&-2 \end{array}[/latex]
 2″ width=”300″ height=”70″ class=”alignnone wp-image-634 size-medium”>
2″ width=”300″ height=”70″ class=”alignnone wp-image-634 size-medium”> - [latex]\begin{array}{rrrrrrrrrrr} \\ \\ \\ 2x&-&3&<&-5&\hspace{0.5in}&2x&-&3&>&5 \\ &+&3&&+3&\hspace{0.5in}&&+&3&&+3 \\ \hline &&2x&<&-2&\hspace{0.5in}&&&2x&>&8 \\ &&x&<&-1&\hspace{0.5in}&&&x&>&4 \end{array}[/latex]
 -1, x < 4" width="300" height="65" class="alignnone wp-image-635 size-medium">
-1, x < 4" width="300" height="65" class="alignnone wp-image-635 size-medium"> -
[latex]y=|x|-3[/latex] [latex]x[/latex] [latex]y[/latex] 3 0 2 −1 1 −2 0 −3 −1 −2 −2 −1 −3 0 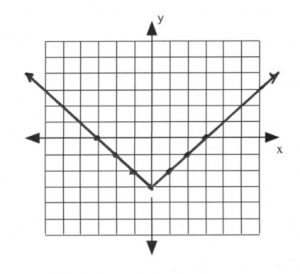
- [latex]\begin{array}{ll} \\ \\ \\ \\ \\ \\ \\ \begin{array}{rrrrl} 5L&+&3S&=&49 \\ 4L&-&2S&=&26 \\ \\ \dfrac{4L}{2}&-&\dfrac{2S}{2}&=&\dfrac{26}{2} \\ \\ 2L&-&S&=&13 \\ \\ &\text{or}&S&=&2L-13 \end{array} & \hspace{0.25in} \begin{array}{rrcrrrl} \\ \\ \\ 5L&+&3(2L&-&13)&=&49 \\ 5L&+&6L&-&39&=&49 \\ &&&+&39&&+39 \\ \hline &&&&\dfrac{11L}{11}&=&\dfrac{88}{11} \\ \\ &&&&L&=&8 \\ \\ &&&&\therefore S&=&2L-13 \\ &&&&S&=&2(8)-13 \\ &&&&S&=&16-13 \\ &&&&S&=&3 \end{array} \end{array}[/latex]
- [latex]\begin{array}{rrl} \\ \\ \\ \\ \\ 5x+x&=&42 \\ 6x&=&42 \\ x&=&\dfrac{42}{6}\text{ or }7 \\ \\ \therefore 5x&=&5(7)\text{ or }35 \end{array}[/latex]
- [latex]\begin{array}{ll} \\ \\ \\ \\ \\ \\ \\ \\ \\ \begin{array}{rrl} \\ \\ \\ \\ \\ \\ \\ y&=&\dfrac{km}{d^2} \\ \\ &&\textbf{1st} \\ y&=&3 \\ k&=&\text{find 1st} \\ m&=&2 \\ d&=&4 \\ \\ y&=&\dfrac{km}{d^2} \\ \\ 3&=&\dfrac{k(2)}{(4)^2} \\ \\ 3&=&\dfrac{3(4)^2}{2} \\ \\ k&=&24 \end{array} & \hspace{0.25in} \begin{array}{rrl} \\ \\ \\ \\ \\ \\ \\ &&\textbf{2nd} \\ y&=&\text{find} \\ k&=&24 \\ m&=&25 \\ d&=&5 \\ \\ y&=&\dfrac{km}{d^2} \\ \\ y&=&\dfrac{(24)(25)}{(5)^2} \\ \\ y&=&24 \end{array} \end{array}[/latex]

