Final Exam: Version B Answer Key

Questions from Chapters 1 to 3

1. [latex]\begin{array}{l} \\ \\ \\ \\ \\ \\ -2(-3)-\sqrt{(-3)^2-4(4)(-1)} \\ \\ 6-\sqrt{9+16} \\ \\ 6-5 \\ \\ 1 \end{array}[/latex]
2. [latex]\begin{array}{rrrrrcllll} \\ \\ \\ \\ \\ \\ \\ &18x&-&30&=&3[4&-&4x&-&7] \\ &18x&-&30&=&3[-4x&-&3]&& \\ &18x&-&30&=&-12x&-&\phantom{1}9&& \\ +&12x&+&30&&+12x&+&30&& \\ \hline &&&30x&=&21&&&& \\ \\ &&&x&=&\dfrac{21}{30}&=&\dfrac{7}{10}&& \end{array}[/latex]
3. [latex]\phantom{1}[/latex]
   [latex]\left(\dfrac{x+4}{2}-\dfrac{1}{3}=\dfrac{x+2}{6}\right)(6) \\[/latex]
   [latex]\begin{array}{rrcrcrrrr} (x&+&4)(3)&-&1(2)&=&x&+&2 \\ 3x&+&12&-&2&=&x&+&2 \\ -x&-&10&&&&-x&-&10 \\ \hline &&&&\dfrac{2x}{2}&=&\dfrac{-8}{2}&& \\ \\ &&&&x&=&-4&& \end{array}[/latex]
4. [latex]\begin{array}{rrrrrrrrr} \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ &&&&m&=&\dfrac{\Delta y}{\Delta x}&& \\ \\ &&&&\dfrac{2}{3}&=&\dfrac{y-4}{x-1}&& \\ \\ &&2(x&-&1)&=&3(y&-&4) \\ &&2x&-&2&=&3y&-&12 \\ &&-3y&+&12&&-3y&+&12 \\ \hline 2x&-&3y&+&10&=&0&& \\ \\ &&&&y&=&\dfrac{2}{3}x&+&\dfrac{10}{3} \\ \end{array}[/latex]
5. [latex]\begin{array}{rrl} \\ \\ \\ \\ \\ \\ d^2&=&\Delta x^2+\Delta y^2 \\ &=&(4--4)^2+(4--2)^2 \\ &=&8^2+6^2 \\ &=&64+36 \\ &=&100 \\ \\ d&=&10 \end{array}[/latex]

6. [latex]3x-2y=6[/latex]

   [latex]x[/latex]	[latex]y[/latex]
   2	0
   0	−3
   −2	−6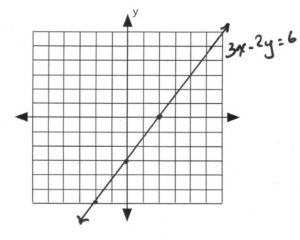

   

7. [latex]\begin{array}{rrrcrrr} \\ \\ \\ \\ \\ 3&\le &6x&+&3&<&9 \\ -3&&&-&3&&-3 \\ \hline \dfrac{0}{6}&\le &&\dfrac{6x}{6}&&<&\dfrac{6}{6} \\ \\ 0&\le &&x&&<&1 \end{array}[/latex] [latex]\phantom{1}[/latex] [latex](0,1)[/latex]
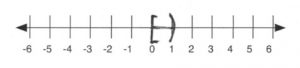
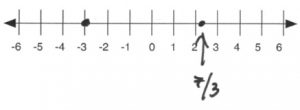
8. [latex]\begin{array}{ll} \\ \\ \\ \\ \\ \\ \\ \\ \left(\dfrac{3x+1}{4}=2\right)^4 & \hspace{0.25in} \left(\dfrac{3x+1}{4}=-2\right)^4 \\ \begin{array}{rrrrr} \\ \\ 3x&+&1&=&8 \\ &-&1&&-1 \\ \hline &&3x&=&7 \\ \\ &&x&=&\dfrac{7}{3} \end{array} & \hspace{0.25in} \begin{array}{rrrrr} 3x&+&1&=&-8 \\ &-&1&&-1 \\ \hline &&3x&=&-9 \\ &&x&=&-3 \end{array} \end{array}[/latex]
   
9. [latex]\begin{array}{ll} \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \hspace{0.75in} w_{\text{m}}=kw_e \\ \begin{array}{rrl} \\ \\ \\ &&\text{1st data} \\ \\ w_{\text{m}}&=&38\text{ lb} \\ k&=&\text{find 1st} \\ w_{\text{e}}&=&95\text{ lb} \\ \\ w_{\text{m}}&=&kw_{\text{e}} \\ 38&=&k(95) \\ \\ k&=&\dfrac{38}{95} \\ \\ k&=&0.4 \end{array} & \hspace{0.25in} \begin{array}{rrl} &&\text{2nd data} \\ \\ w_{\text{m}}&=&\text{find} \\ k&=&0.4 \\ w_{\text{e}}&=&240\text{ lb} \\ \\ w_{\text{m}}&=&kw_{\text{e}} \\ w_{\text{m}}&=&(0.4)(240) \\ w_{\text{m}}&=&96\text{ lb} \end{array} \end{array}[/latex]
10. [latex]\phantom{1}[/latex]
    [latex]x, x+2 \\[/latex]
    [latex]\begin{array}{rrrrrrrrrrr} x&+&x&+&2&=&x&+&2&-&20 \\ &&2x&+&2&=&x&-&18&& \\ &&-x&-&2&&-x&-&2&& \\ \hline &&&&x&=&-20&&&& \end{array}[/latex]
    [latex]\phantom{1}[/latex]
    [latex]\text{numbers are }-20, -18[/latex]

Questions from Chapters 4 to 6

1. [latex]\begin{array}{rrrrrl} \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ &(4x&-&3y&=&13)(5) \\ &(6x&+&5y&=&-9)(3) \\ \\ &20x&-&15y&=&\phantom{-}65 \\ +&18x&+&15y&=&-27 \\ \hline &&&38x&=&38 \\ &&&x&=&1 \\ \\ &4(1)&-&3y&=&13 \\ &-4&&&&-4 \\ \hline &&&-3y&=&9 \\ &&&y&=&-3 \end{array}[/latex]
   [latex](1,-3)[/latex]
2. [latex]\begin{array}{rrrrrrl} \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ &&&&x&=&-1-y \\ \\ \therefore 3(-1&-&y)&-&4y&=&-5 \\ -3&-&3y&-&4y&=&-5 \\ +3&&&&&&+3 \\ \hline &&&&-7y&=&-2 \\ &&&&y&=&\dfrac{2}{7} \\ \\ &&x&+&y&=&-1 \\ &&x&+&\dfrac{2}{7}&=&-1 \\ \\ &&&-&\dfrac{2}{7}&&-\dfrac{2}{7} \\ \hline &&&&x&=&-\dfrac{9}{7} \end{array}[/latex]
3. [latex]\begin{array}{rrrrrrrl} \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ &&&(x&-&4z&=&0)(-1) \\ \\ &x&+&2y&&&=&0 \\ +&-x&&&+&4z&=&0 \\ \hline &&&(2y&+&4z&=&0)(\div 2) \\ &&&y&+&2z&=&0 \\ \\ &&&y&+&2z&=&0 \\ &&+&y&-&2z&=&0 \\ \hline &&&&&2y&=&0 \\ &&&&&y&=&0 \\ \\ &&&\cancel{y}0&-&2z&=&0 \\ &&&&&z&=&0 \\ \\ &&&x&+&\cancel{2y}0&=&0 \\ &&&&&x&=&0 \end{array}[/latex]
   [latex](0,0,0)[/latex]
4. [latex]\begin{array}{l} \\ \\ \\ 28-\{5\cancel{x^0}1-\cancel{\left[6x-3(5-2x)\right]^0}1\}+5\cancel{x^0}1 \\ 28-\{5-1\}+5 \\ 28-4+5 \\ 29 \end{array}[/latex]
5. [latex]\begin{array}{rrrrrrrr} \\ \\ \\ \\ \\ &x^2&-&3x&+&8\phantom{x}&& \\ \times&&&x&-&4\phantom{x}&& \\ \hline &x^3&-&3x^2&+&8x&& \\ +&&-&4x^2&+&12x&-&32 \\ \hline &x^3&-&7x^2&+&20x&-&32 \\ \end{array}[/latex]
6. [latex]\begin{array}{l} \\ \\ (x^{3n-6-3n})^{-1} \\ (x^{-6})^{-1} \\ x^6 \end{array}[/latex]
7. [latex]5y(5y^2-3y+1)[/latex]
8. [latex]\begin{array}{l} \\ x^3+(2y)^3 \\ (x+2y)(x^2-2xy+4y^2) \end{array}[/latex]

9. Solution	Amount	Strength	Equation
   Soda	[latex]x[/latex]	0	0
   Juice	2	35	2 (35)
   Diluted	[latex]x+2[/latex]	8	[latex](x+2)8[/latex]

   [latex]\begin{array}{rrrrrl} &2(35)&=&8(x&+&2) \\ &70&=&8x&+&16 \\ -&16&&&-&16 \\ \hline &54&=&8x&& \\ \\ &x&=&\dfrac{54}{8}&\text{ or }&6\dfrac{3}{4}\text{ litres} \\ \end{array}[/latex]

10. [latex]\begin{array}{rrrrrl} \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ &(d&+&q&=&14)(-10) \\ &10d&+&25q&=&185 \\ \\ &-10d&-&10q&=&-140 \\ +&10d&+&25q&=&\phantom{-}185 \\ \hline &&&\dfrac{15q}{15}&=&\dfrac{45}{15} \\ \\ &&&q&=&3 \\ \\ &\therefore d&+&3&=&14 \\ &&&d&=&11 \end{array}[/latex]

Questions from Chapters 7 to 10

1. [latex]\dfrac{\cancel{9}\cancel{s^2}}{7t^3}\cdot \dfrac{15\cancel{t}}{\cancel{13}\cancel{s^2}}\cdot \dfrac{\cancel{26}2s}{\cancel{9}\cancel{t}}\Rightarrow \dfrac{15\cdot 2\cdot 5}{7t^3}\Rightarrow \dfrac{30s}{7t^3}[/latex]
2. [latex]\begin{array}{l} \\ \\ \\ \\ \\ \\ \dfrac{(a-1)2a}{(a-1)(a-6)(a+6)}-\dfrac{5(a+6)}{(a-6)(a-1)(a+6)} \Rightarrow \dfrac{2a^2-2a-5a-30}{(a-1)(a-6)(a+6)} \\ \\ \Rightarrow \dfrac{2a^2-7a-30}{(a-1)(a-6)(a+6)}\Rightarrow \dfrac{2a^2-12a+5a-30}{(a-1)(a-6)(a+6)} \\ \\ \Rightarrow \dfrac{2a(a-6)+5(a-6)}{(a-1)(a-6)(a+6)}\Rightarrow \dfrac{\cancel{(a-6)}(2a+5)}{(a-1)\cancel{(a-6)}(a+6)}\Rightarrow \dfrac{2a+5}{(a-1)(a+6)} \end{array}[/latex]
3. [latex]\dfrac{\left(1-\dfrac{8}{x}\right)x^2}{\left(\dfrac{3}{x}-\dfrac{24}{x^2}\right)x^2}\Rightarrow \dfrac{x^2-8x}{3x-24}\Rightarrow \dfrac{x\cancel{(x-8)}}{3\cancel{(x-8)}}\Rightarrow \dfrac{x}{3}[/latex]
4. [latex]\begin{array}{l} \\ \\ \\ \\ \sqrt{x^4\cdot x\cdot y^6\cdot y}+2xy\sqrt{16\cdot x\cdot y^2\cdot y}-\sqrt{x\cdot y^2\cdot y} \\ \\ x^2y^3\sqrt{xy}+2xy\cdot 4y\sqrt{xy}-y\sqrt{xy} \\ \\ (x^2y^3+8xy^2-y)\sqrt{xy} \end{array}[/latex]
5. [latex]\dfrac{2+x}{1-\sqrt{7}}\cdot \dfrac{1+\sqrt{7}}{1+\sqrt{7}}\Rightarrow \dfrac{2+2\sqrt{7}+x+x\sqrt{7}}{1-7}\Rightarrow \dfrac{2+x+2\sqrt{7}+x\sqrt{7}}{-6}[/latex]
6. [latex]\left(\dfrac{a^6b^3}{\cancel{c^0}1d^{-9}}\right)^{\frac{2}{3}}\Rightarrow \dfrac{a^{6\cdot \frac{2}{3}}b^{3\cdot \frac{2}{3}}}{d^{-9\cdot \frac{2}{3}}}\Rightarrow \dfrac{a^4b^2}{d^{-6}}\Rightarrow a^4b^2d^6[/latex]
7. [latex]\begin{array}{rrl} \\ (x-5)(x+3)&=&0 \\ x&=&5, -3 \end{array}[/latex]
8. [latex]\phantom{1}[/latex]
   [latex]\left(\dfrac{2x-1}{3x}=\dfrac{x-3}{x}\right)(3x) \\[/latex]
   [latex]\begin{array}{rrrrrrrr} &2x&-&1&=&(x&-&3)(3) \\ \\ &2x&-&1&=&3x&-&9\phantom{)(3)} \\ +&-3x&+&1&&-3x&+&1\phantom{)(3)} \\ \hline &&&-x&=&-8&& \\ &&&x&=&8&& \end{array}[/latex]
9. [latex]\begin{array}{rrl} \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ \\ A&=&L\cdot W \\ L&=&5+2W \\ \\ 75&=&W(5+2W) \\ 75&=&5W+2W^2 \\ \\ 0&=&2W^2+5W-75 \\ 0&=&2W^2-10W+15W-75 \\ 0&=&2W(W-5)+15(W-5) \\ 0&=&(W-5)(2W+15) \\ W&=&5, \cancel{-\dfrac{15}{2}} \\ \\ L&=&5+2(5) \\ L&=&15 \end{array}[/latex]
10. [latex]\phantom{1}[/latex]
    [latex]x, x+2, x+4 \\[/latex]
    [latex]\begin{array}{rrcrrrrrrrr} &&x(x&+&2)&=&8(x&+&4)&-&25 \\ x^2&+&2x&&&=&8x&+&32&-&25 \\ &-&8x&-&32&&-8x&-&32&+&25 \\ &&&+&25&&&&&& \\ \hline x^2&-&6x&-&7&=&0&&&& \\ (x&-&7)(x&+&1)&=&0&&&& \\ &&&&x&=&7,&-1&&& \\ \end{array}[/latex]
    [latex]\phantom{1}[/latex]
    [latex]\text{numbers are }7,9,11\text{ or }-1,1,3[/latex]